GoldenRatioUltra

The Golden Ratio is essential to what we consider beautiful. Anything close to the golden ratio, approximately 1.618, is more beautiful to the human eye than something that is not. The Golden Ratio is represented by the Greek symbol φ

Throughout history, the Golden Ratio has been used in both art and architecture.

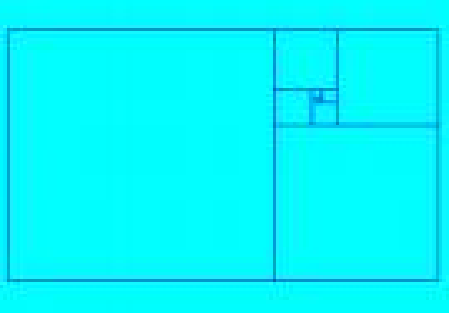
Below is an image of the Golden Rectangle. It is basically a rectangle divided into two perfect halves, with one of these halves divided in half itself, and so on. Even though it is not based on the Golden Ratio, European paper format follows much the same pattern (A3, A4, etc.)

The Golden Ratio is explained in the problem below, which algebraically demonstrates the geometrical ratio between the length (b) and width (a) of a rectangle that makes it a so called golden rectangle. The ratio of the length to the width of the original rectangle is equal to the ratio of the length and width of a new rectangle where the new length is the old length + the old width and the new width is the former length.

(a+b)/b=b/a=golden ratio=φ

(a+b)/b = a/b +1 = 1/φ+1 = φ

1+φ=φ²

As we will learn later, G = (1+ √5) / 2